Dx x xx 1 5. Since u = 1−x2, x2 = 1− u and the integral is z − 1 2 (1−u) √ udu.
Integration Rules With Examples Pdf. Alternative form of log rule example 1 using the log rule for integration constant multiple rule log rule for integration property of logarithms because cannot be negative, the absolute value is unnecessary in the final form of the antiderivative. Basic integration rules using integration definition.
Ayusya Home Health Care Pvt LtdBangaloreChennaiMadurai From ayusyahomehealthcare.com
Since u = 1−x2, x2 = 1− u and the integral is z − 1 2 (1−u) √ udu. For example, if the problem is to find (7.14) xcosxdx then we can easily differentiate f x x, and integrate cosxdx separately. Sometimes you have to integrate powers of secant and tangents too.
Ayusya Home Health Care Pvt LtdBangaloreChennaiMadurai
Example 2 using the log rule with a change of variables find solution if you let then multiply and divide by 4. Substituting u = x−1 and du = dx,youget z £ (x−1)5 +3(x−1) 2+5 ¤ dx = z (u5 +3u +5)du = = 1 6 u6 +u3 +5u+c = = 1 6 Integral = z 2 y=1 z 3 x=0 (1+8xy)dx | {z } ( 2 3)x x dx 2 23 8 5 6 4.
Source: venturebeat.com
8 140000 2100 140000 2000ln t. 7.1.3 geometrically, the statement∫f dx()x = f (x) + c = y (say) represents a family of. U =sin x (trig function) (making “same” choices for u and dv) dv =ex dx (exponential function) du =cosx dx v =∫ex dx =ex ∫ f dx + ∫ g dx: Starting with (3, 12) and using.
Source: pinterest.com
∫ f dx + ∫ g dx: Basic integration rules using integration definition. ∫ex cosx dx u =cos x (trig function) dv =ex dx (exponential function) du =−sin x dx v =∫ex dx =ex ∫ex cosx dx =uv−∫vdu =cosx ex −∫ex (−sin x) dx =cosx ex +∫ex sin x dx second application of integration by parts: Integral = z 2.
Source: ayusyahomehealthcare.com
The same is true of our current expression: ( ) 3 x dx Substituting u = x−1 and du = dx,youget z £ (x−1)5 +3(x−1) 2+5 ¤ dx = z (u5 +3u +5)du = = 1 6 u6 +u3 +5u+c = = 1 6 If n6= 1 lnjxj+ c; < xn = b, and xi ¡ xi¡1 = h =.
Source: ayusyahomehealthcare.com
F x e x3 ln , 1,0 example: We used basic antidifferentiation techniques to find integration rules. C) find the absolute relative true error for part (a). For example, if the problem is to find (7.14) xcosxdx then we can easily differentiate f x x, and integrate cosxdx separately. 2h f(x0)+4f(x1)+f(x2) 6 +2h f(x2)+4f(x3)+f(x4) 6 +¢¢¢+2h
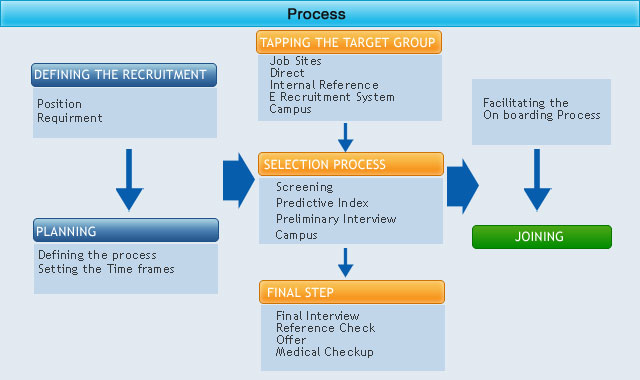Source: examples.com
Multiply and divide by 2. 8 140000 2100 140000 2000ln t. Consider, forexample, the chain rule. ( 2 3)x x dx 2 23 8 5 6 4. Power rule (n≠−1) ∫ x n dx:
Source: thewaythetruthandthelife.net
2h f(x0)+4f(x1)+f(x2) 6 +2h f(x2)+4f(x3)+f(x4) 6 +¢¢¢+2h If n= 1 exponential functions with base a: Example 1 the vertical distance covered by a rocket from. We interpreted constant of integration graphically. C) find the absolute relative true error for part (a).
