44+ Integration Of Log Tanx Dx

2) on differentiating it with respect to x you get. Let i = `int_0^ (pi/2) log (tanx)*dx`.

Integration Of Log Tanx Dx. He has been teaching from the past 10 years. 2) on differentiating it with respect to x you get.

Integral cosx ln cos x Matematicas 2º Bachillerato AINTE From youtube.com

I=int1/((1+u^2)(1+u))du apply partial fraction decomposition: Let u=log (secx+tanx) differentiate wrt x. Ex 7.11, 8 by using the properties of definite integrals, evaluate the integrals :

isolation thermique exterieur prix interrupteur design pas cher indigo airlines cargo contact number delhi joint silicone gris

Integral cosx ln cos x Matematicas 2º Bachillerato AINTE

Let u=log (secx+tanx) differentiate wrt x. (a) π/4 (b) π/2 (c) 0 (d) π. Integral 0 to pi/2 of sin 2x log tanx dx. \begin{align}i&=\int\ln(\tan x)\mathrm dx\\hline&\text{let }u=\tan x\implies \mathrm du=\sec^2x,\mathrm dx.

Source: physicsforums.com

Post your answer (best answer will be rewarded with handsome gifts) please login or register for upload image. Let u=log (secx+tanx) differentiate wrt x. Let i = int_0^ (pi/2) log (tanx)*dx. I=int1/((1+u^2)(1+u))du apply partial fraction decomposition: ⇒ i = ∫π/4 0 log(1+tan[π 4 −x]dx i = ∫ 0 π / 4 l o g ( 1 + t a n.

Source: thewaythetruthandthelife.net

Let i = int_0^ (pi/2) log (tanx)*dx. ⇒ ∫a 0 f(x)dx= ∫a 0 f(a−x)dx ∫ 0 a f ( x) d x = ∫ 0 a f ( a − x) d x. I=int1/((1+u^2)(1+u))du apply partial fraction decomposition: Let i = (\int_{0}^{\pi/4}) log(1 + tan x) dx. 1 + ( 1 secx ⋅ secx.tanx) = dt dx.

Source: teachoo.com

Let i = ∫ cos 2 x. We perform this integration like this. He has been teaching from the past 10 years. Du/dx = {1/ (secx+tanx)} * { d (secx)/dx + d (tanx)/dx} du/dx = {1/ (secx+tanx)} * { (secx*tanx)+ (sec2x)} du/dx = {1/ (secx+tanx)} * { (tanx)+ (secx)} * secx. I = ∫π/4 0 log(1+tanx)dx i = ∫ 0.

Source: teachoo.com

Dx let us consider log(sinx) = z cosx/sinx = z → ∫zdz = z²/2 + c hope it helps you ! The value of the integral ∫ log tan x dx x ∈ [0,π ⁄ 2] is equal to : I = ( [log (secx+tanx)] 2/2) + c. We perform this integration like this. Where c is the integration constant.

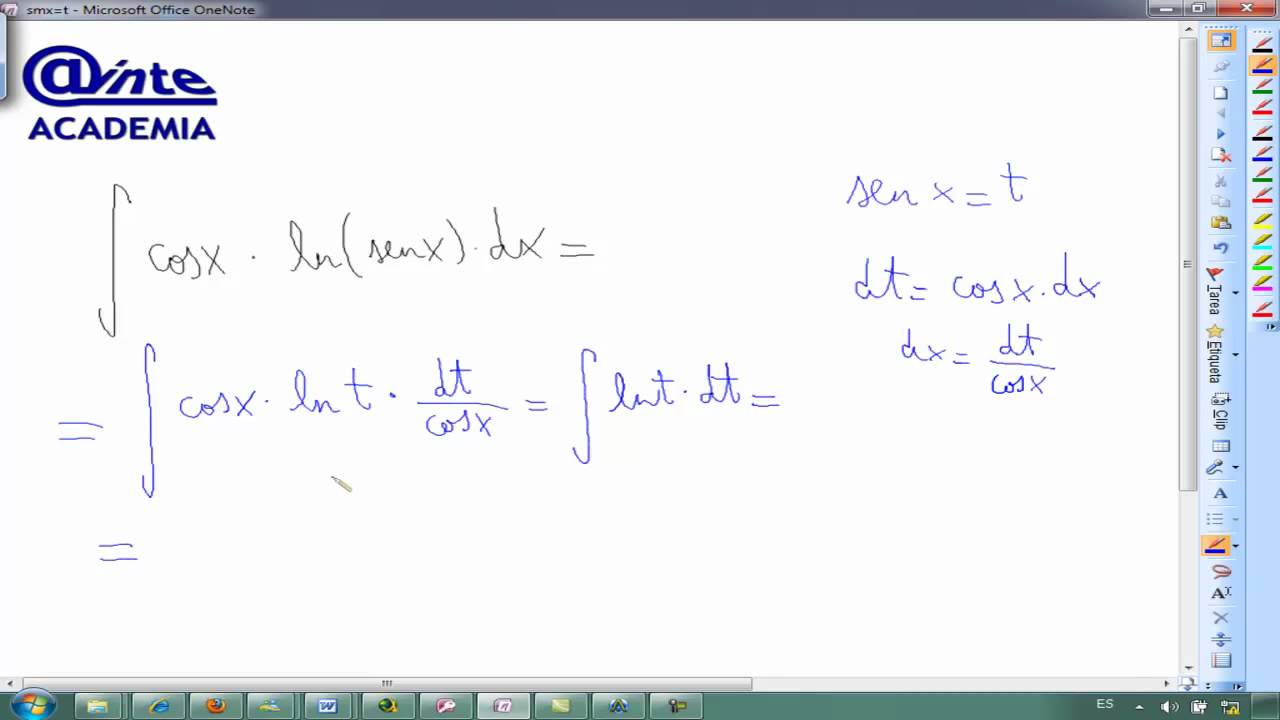Source: youtube.com

Join / login >> class 12 >> maths >> integrals >> integration by parts >> the value of int log x dx is | maths que. ∫sin 2x log(tan x) dx for x ∈ [0,π/2]. ∫_0^(𝜋/4) log⁡(1+tan⁡𝑥 ) 𝑑𝑥 let i=∫_0^(𝜋/4) log⁡〖 (1+tan⁡𝑥 )〗 𝑑𝑥 ∴ i=∫_0^(𝜋/4) log⁡[1+tan⁡(𝜋/4−𝑥) ] 𝑑𝑥 i=∫_0^(𝜋/4) log⁡[1+(tan⁡ 𝜋/4 −tan⁡𝑥)/(1 +〖 tan〗⁡ 𝜋/4. Post your answer.