U sin 1 x (inverse trig function) dv 1 dx. Z ˇ=4 0 sec3 d = 1 2 (sec tan +lnjsec +tan j) ˇ=4 0 = 1 2 p 2+ln(p.
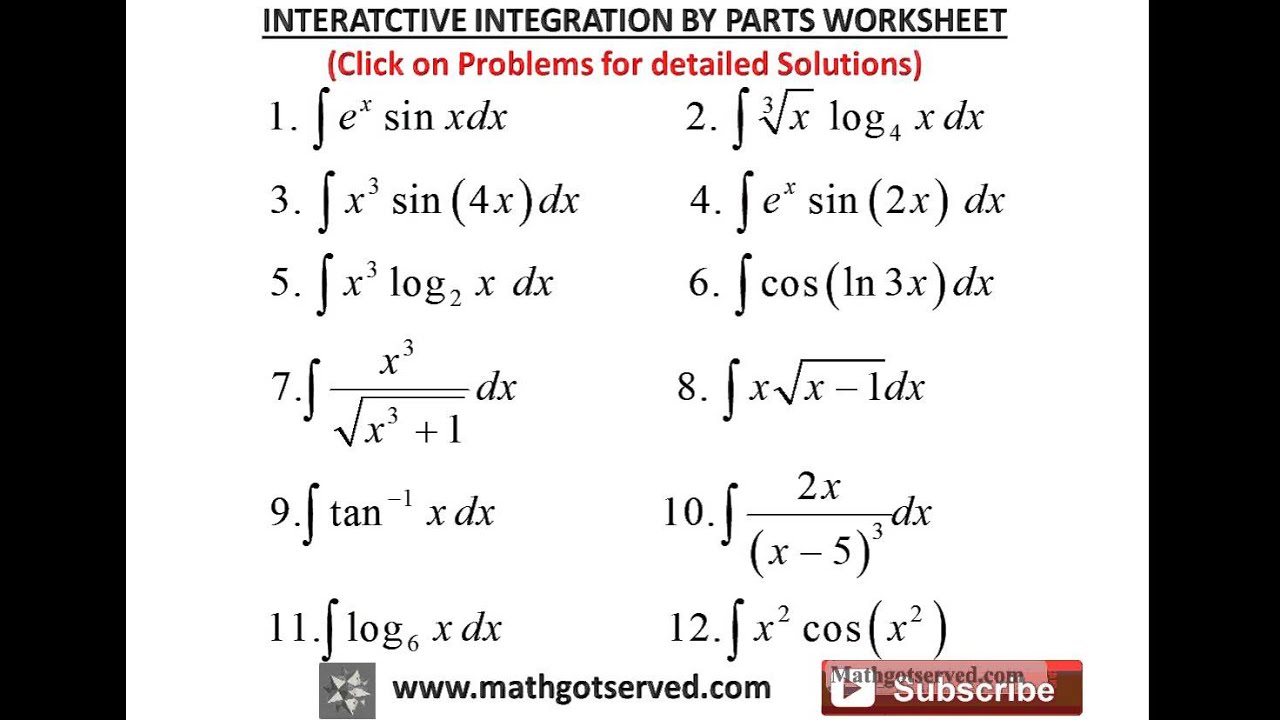
Integration By Parts Practice Problems. I pick the representive ones out. Here are a few things to keep in mind while working.
integration by parts interactive worksheet YouTube From youtube.com
= =(=() + =() + + =() + + (() =′() = =) = = + = =() = (() + + (. We want to choose u u and d v d v so that when we compute d u d u and v v and plugging everything into the integration by parts formula the new integral we get is one that we can do. With that in mind it looks like the following choices for u u and d v d v should work for us.
integration by parts interactive worksheet YouTube
The method of integration by parts all of the following problems use the method of integration by parts. For example, if , then the differential of is Let u= sinx, dv= exdx. ∫ x 3 ln x d x.
Source: venturebeat.com
Let u= sinx, dv= exdx. •for question 4 put x4=u and then solve. The following are solutions to the trig substitution practice problems posted on november 9. The following are solutions to the integration by parts practice problems posted november 9. Then z exsinxdx= exsinx excosx z exsinxdx
Source: youtube.com
Integration by parts practice problems. What to watch out for. Plugging u u, d u d u, v v and d v d v into the integration by parts formula gives, ∫ t 7 sin ( 2 t 4) d t = − 1 8 t 4 cos ( 2 t 4) + 1 2 ∫ t 3 cos (.
Source: venturebeat.com
This chapter is the start of more challenging integration problems. With that in mind it looks like the following choices for u u and d v d v should work for us. Then du= cosxdxand v= ex. Evaluate ∫ (3t+t2)sin(2t)dt ∫ ( 3 t + t 2) sin. We want to choose u u and d v d v so.
Source: venturebeat.com
Integration by partial fractions summary: (6) x2 e 2x solution. Let x−r be a linear factor of g(x). Important tips for practice problem •if you see a function and its derivative put function=u e.g. The method of integration by parts all of the following problems use the method of integration by parts.
