You will see plenty of examples soon, but first let us see the rule: When the product of two functions is presented to us, we use the needed formula, as.
Integration By Parts Ilate Rule. In which the integrand is the product of two functions can be solved using integration by parts. Ilate rule is used in integration when we are doing integration by parts i.e when there is product of two functions and we have to integrate it.
11. Integration by Parts Proving Reduction Formula YouTube From youtube.com
A useful rule of integral by parts is ilate. Normally we use the preference order for the first function i.e. Then we�ll take first function to be.
11. Integration by Parts Proving Reduction Formula YouTube
The liate method was rst mentioned by herbert e. What is integration by parts formula. This rule helps us determine which function should be treated as the first function of x that is u (x) and which function should be referred to. Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways.
Source: youtube.com
The di culty of integration by parts is in choosing u(x) and v0(x) correctly. By adopting the left term as the first function and the second term as the second function, the integral of the two functions is obtained. When the product of two functions is presented to us, we use the needed formula, as we learned in integration by.
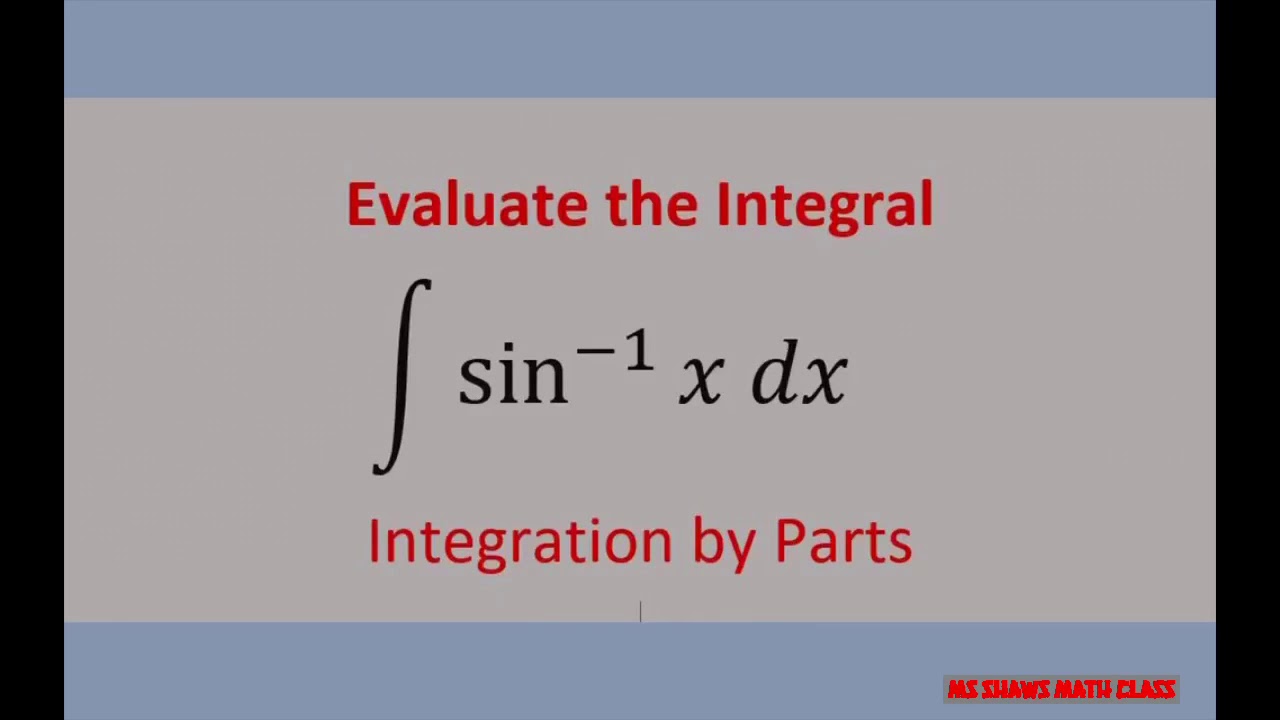Source: youtube.com
Using repeated applications of integration by parts: Tabular integration by parts when integration by parts is needed more than once you are actually doing integration by parts recursively. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more easily found. The ilate rule helps us make use.
Source: youtube.com
Then we�ll take first function to be. Ilate rule (inverse, logarithmic, algebraic, trigonometric, exponent) which states that the inverse function should be assumed as the first function while performing the integration. It is frequently used to transform the antiderivative of a product of functions into an antiderivative for which a solution can be more easily found. U is the function.
Source: slideshare.net
The ilate rule helps us make use of it in the correct way. As if there is two functions. ∫x2 sin x dx u =x2 (algebraic function) dv =sin x dx (trig function) du =2x dx v =∫sin x dx =−cosx ∫x2 sin x dx =uv−∫vdu =x2 (−cosx) − ∫−cosx 2x dx =−x2 cosx+2 ∫x cosx dx second application of.
Source: youtube.com
Ilate rule (inverse, logarithmic, algebraic, trigonometric, exponent) which states that the inverse function should be assumed as the first function while performing the integration. This rule states that the inverse trigonometric function should be assumed as the first function while. The di culty of integration by parts is in choosing u(x) and v0(x) correctly. Integration by parts is a procedure.
Source: teachoo.com
The ilate rule helps us make use of it in the correct way. You will see plenty of examples soon, but first let us see the rule: The di culty of integration by parts is in choosing u(x) and v0(x) correctly. Then the product rule in. ∫ u v dx = u ∫ v dx − ∫ u� ( ∫.
