49++ Integral Rules Ln

Integratingln ⁡ xln x lnx. How do you know if a linear approximati….

Integral Rules Ln. Ln (x) dx = u dv. Integral of natural log ln(x) the general rule for the integral of natural log is:

Calculus cheat sheet_integrals From slideshare.net

C c will be used throughout the wiki. Z ax dx= ax ln(a) + c with base e, this becomes: Integratingln ⁡ x\ln x lnx.

joint pour porte fenetre pvc honda integra dc5 type r spec interrupteur arnould 2001 indigo flight ticket printout

Calculus cheat sheet_integrals

(b) the integral of y = x nis z x dx = x(n+1) (n +1), for n 6= −1. ∫ (1 / 2) ln (x) dx = (1 / 2) ∫ ln (x) dx we now use formula 4.3 in the table of integral formulas to evaluate ∫ ln (x) dx. Learn your rules (power rule, trig rules, log rules, etc.). So if the function we are trying to integrate is a quotient, and if the numerator is the derivative of the denominator, then the integral will involve a logarithm:

Source: youtube.com

In the equation above, c. So if the function we are trying to integrate is a quotient, and if the numerator is the derivative of the denominator, then the integral will involve a logarithm: Z ax dx= ax ln(a) + c with base e, this becomes: Integral of natural logarithm (ln) function. C c is the constant of integration, and.

Source: philschatz.com

Integratingln ⁡ x\ln x lnx. Theorem 5.5 log rule for integration let be a differentiable function of 1. Integral of natural logarithm (ln) function. Must know derivative and integral rules! So if the function we are trying to integrate is a quotient, and if the numerator is the derivative of the denominator, then the integral will involve a logarithm:

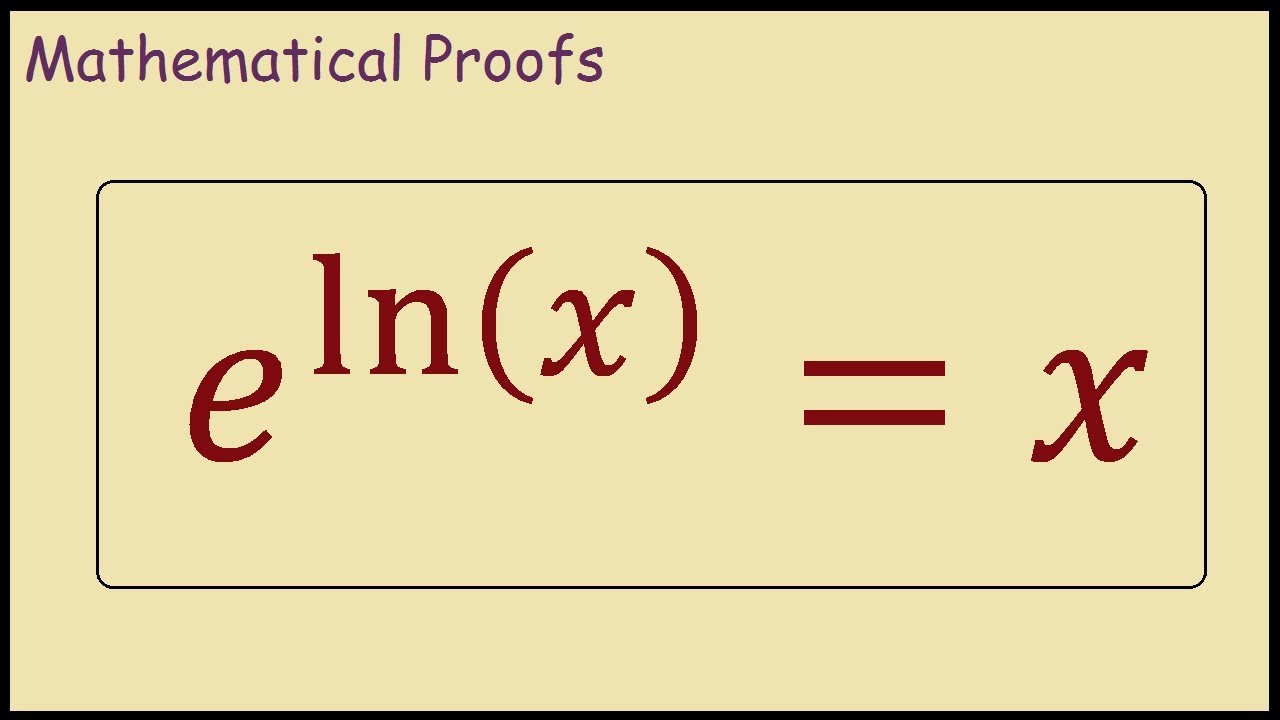Source: youtube.com

For this solution, we will use integration by parts: = ln + c d dx log b jx = 1 xlnb same as above d dx ex= r dx + c d dx bx= (ln ) r dx = 1 lnb bx+ c d dx sin( x) = cos( ) r cos( dx. We can approximate integrals using riemann sums,.

Source: slideshare.net

Exploration integrating rational functions early in chapter 4, you learned rules that allowed you to integrate polynomial function. Z ax dx= ax ln(a) + c with base e, this becomes: Definition as an integral recall: Substitute u=ln (x), v=x, and du= (1/x)dx. (b) the integral of y = x nis z x dx = x(n+1) (n +1), for n 6=.