The first step we need to take is to learn how to make the inverse operation to differentiation, called finding antiderivatives: We consent this nice of integral calculus examples graphic.
Integral Calculus Definition And Example. Definitions and meaning of integral calculus in english integral calculus noun. Example 2 evaluate each of the following.
Desmos A Definitive Guide on Graphing and Computing From mathvault.ca
As with derivatives, integrals generalize a simple intuitive concept that we use everyday: To calculate the area under a curve. Definitions and meaning of integral calculus in english integral calculus noun.
Desmos A Definitive Guide on Graphing and Computing
It is mostly useful for the following two purposes: 1 6 x6 +c 2. It stresses the inverse relationship between differentiation and integration. (c) at what speed is the car traveling when the brakes are applied if the stopping distance is 56 meters?
Source: maaz.ihmc.us
2x 3 2 + 1 Given the derivative f’ of the function f, we can determine the function f. Examples of working out integrals. Together they form the pair of concepts calculus is all about. To calculate the area under a curve.
Source: mathemania.com
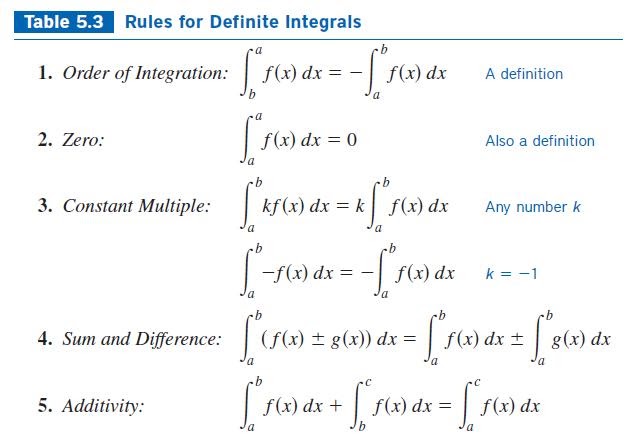
∫ 2 0 x2+1dx ∫ 0 2 x 2 + 1 d x. We identified it from trustworthy source. ∫ 0 4 √t(t−2) dt ∫ 4 0 t ( t − 2) d t. 2 3 x3 2 −9 5 x5 3 +6x+ c 6. ∫ [f (x) − g (x)]dx = ∫f (x)dx − ∫g (x)dx.
Source: youtube.com
∫ 1 −3 6x2 −5x +2dx ∫ − 3 1 6 x 2 − 5 x + 2 d x. ∫ 2 0 x2+1dx ∫ 0 2 x 2 + 1 d x. ∫ xndx = x n + 1 ⁄(n + 1) + c , where n ≠ 0 note though, that as you’re finding a definite integral (as.
Source: flintapcalcbc09.blogspot.com
As with derivatives, integrals generalize a simple intuitive concept that we use everyday: 2x 3 2 + 1 As shown in the last part of this example we can do some fairly complicated looking quotients at this point if we remember to do simplifications when we see them. We identified it from trustworthy source. Examples of working out integrals.
Source: mathvault.ca
Let’s work some more examples. Given the derivative f’ of the function f, we can determine the function f. The part of calculus that deals with integration and its application in the solution of differential equations and in determining areas or volumes etc. The first step we need to take is to learn how to make the inverse operation to.
Source: teachoo.com
4 7 x7 4 +c 3. The part of calculus that deals with integration and its application in the solution of differential equations and in determining areas or volumes etc. 2x 3 2 + 1 As shown in the last part of this example we can do some fairly complicated looking quotients at this point if we remember to do.
