Average & instantaneous velocity x 1 x 2 x 3 2 1 2 1 t t x x vavg − − = 3 2 3 2 t t x vavg.
Instantaneous Velocity And Acceleration. V = 2 t 2. Find the functional form of the acceleration.
Instantaneous Velocity, Acceleration, Jerk, Slopes, Graphs From youtube.com
This is our definition of acceleration. Instantaneous acceleration a(t) is a continuous function of time and gives the acceleration at any specific time during the motion. There is also average acceleration which equals a → = δ v → δ t, over some time δ t.
Instantaneous Velocity, Acceleration, Jerk, Slopes, Graphs
There is also average acceleration which equals a → = δ v → δ t, over some time δ t. Instantaneous velocity at t = 5 sec = (12×5 + 2) = 62 m/s. In a similar manner from our lesson on velocity graphs, we�ll look at the slope of the line in each region. So, if we have to find out the instantaneous velocity at t = 5 sec, then we will put the value of t in the obtained expression of velocity.
Source: slideshare.net
Let us calculate the average velocity now for 5 seconds now. Let�s say that we want to find the acceleration of the particle at the instant t = 3 s. Speed at time t = lim t!0 js(t+ t) s(t)j t = js0(t)j= jv(t)j; The instantaneous velocity at time t. If applicable, the method of instantaneous center can be used.
Source: slideshare.net
Find the instantaneous velocity at t = 1, 2, 3, and 5 s. V = 2 t 2. This is our definition of acceleration. Instantaneous velocity at t = 5 sec = (12×5 + 2) = 62 m/s. So, if we have to find out the instantaneous velocity at t = 5 sec, then we will put the value of.
Source: physics.stackexchange.com
Here, our slope (rise over run) is the change in velocity divided by the change in time, or d v/ d t. So, if we have to find out the instantaneous velocity at t = 5 sec, then we will put the value of t in the obtained expression of velocity. Some books on biomechanics use the term velocity to.
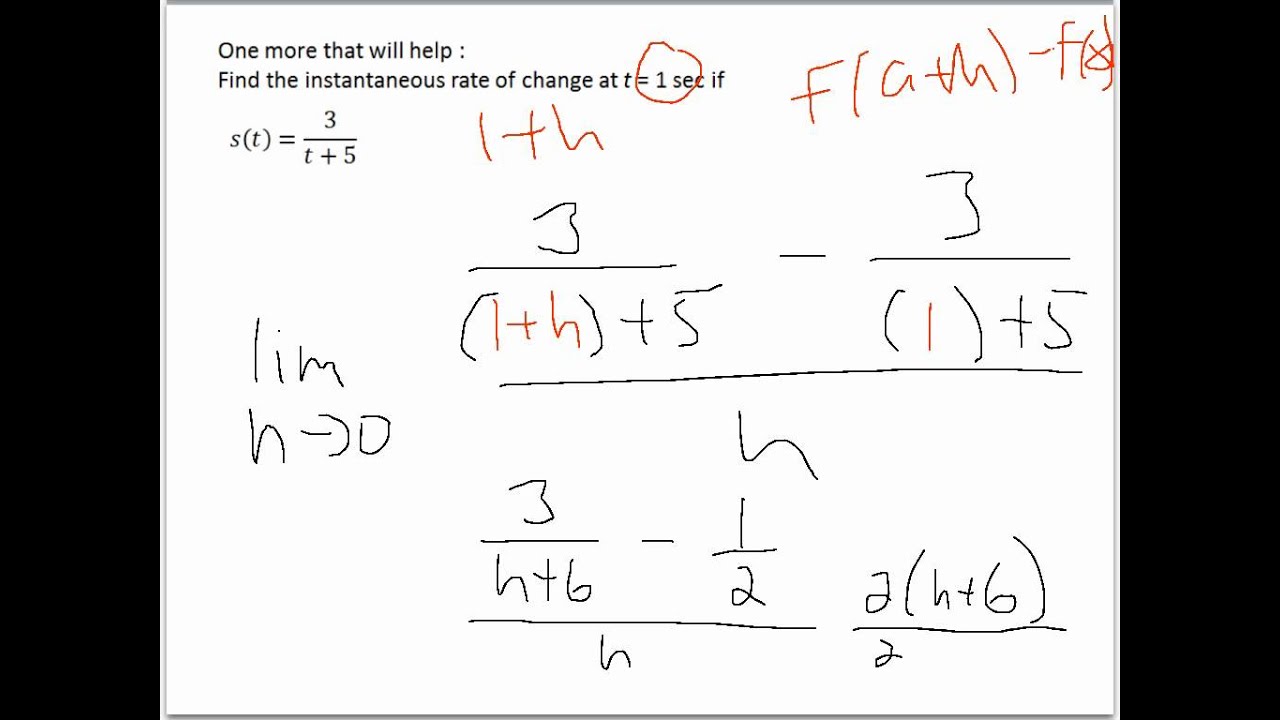Source: youtube.com
The velocity of any point on a rigid body is _____ to the relative position vector extending from the ic. Uniform motion happens when there is no acceleration on the body. V = 2 t 2. Average & instantaneous velocity x 1 x 2 x 3 2 1 2 1 t t x x vavg − − = 3 2.
Source: youtube.com
Above for velocity, we get that instantaneous speed at time t is equal to the absolute value of the instantaneous velocity: Let us calculate the average velocity now for 5 seconds now. Uniform motion happens when there is no acceleration on the body. Find the instantaneous velocity at t = 1, 2, 3, and 5 s. That is, we calculate.
Source: youtube.com
This quantity, the time rate of change of velocity with time, is called the acceleration, and it, too, is a vector. Instantaneous acceleration is a → = d v → d t. Displacement = (6×5 2 + 2×5 + 4) = 164 m. So, if we have to find out the instantaneous velocity at t = 5 sec, then we.
